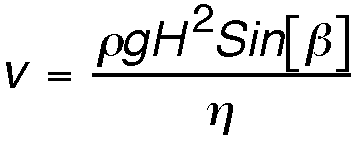Working with equations and plotting in Mathematica
based upon the discussion and demonstration in today's lecture, explore the
following two geologic situations:
1)
The following data came from the Troll 3.1 well in the Norwegian North
Sea:
Depth (cm), Age (years)
19.75, 1490
407, 10510
545, 11160
825, 11730
1158, 12410
1454, 12585
2060, 13445
2263, 14685
Plot a graph of these data, and then using the show command and a function,
plot an "eyeballed" best-fitting straight line through these data.
Then estimate: (i) the sedimentation rate for the last 10,000 years, (ii)
the sedimentation rate for the preceding 5,000 years, (iii) the time
since sedimentation ceased.
(This comes from the book Mathematics: a simple tool for
geologists, by David Waltham, Chapman and Hall, 1995.)
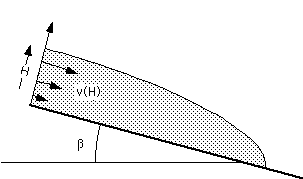
2) As lava flows down the side of a volcano, its velocity , v, is an
important quantity to be able to calculate. The following equation is
found by solving a viscous boundary value problem:
This is a new equation from the morning! this one will work more
realistically!
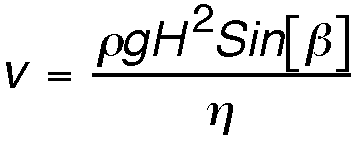
Where
p is the lava density,
g is the acceleration of gravity
H is the lava flow height and the distance measured perpendicular from the earth's surface up
through the flow
beta is the angle of inclination of the slope
eta or whatever it is is hte lava viscosity
Make sure to do some dimensional analysis here!
viscosity has units of ((Force/Area)(time))
(one pascal is a newton/m^2)
(one newton is kg m s^-2)
From Jon Fink:
Typically basalts have been assigned 100 Pa-s. Rhyolites are probably
closer to 10^7-10^9 Pa-s.
Make the best estimate you can for the other parameters (density, slope, etc.)
(i) Plot the velocity distribution of each the flows from the bottom to
the top on separate plots and the same plot. Where is the highest velocity of the flow?
(ii) If you were on the edge of a 5 degree sloping volcano on the
Galapagos (basaltic lava) and were being chased by a 2 m thick lava flow, could you outrun
it?
(iii) If the steepest a volcano slope might get to and still allow for
flow (as opposed to slumping or collapse) is about 30 degrees, how fast
could a rhyolite flow 25 m thick get going?
This problem comes from the so-far unpublished Principles and Practice of
Structural Geology by David Pollard and Arvid Johnson, but the origal
equation was not very satisfactory
Turn in formatted notebooks that solve these geological problems and
answer the questions to the glg490 folder on Caliente public folder and
drop us a line that you have done so at glg490@asu.edu by the beginning of
class on Wednesday, December 10.
Pages maintained by
Prof. Ramón Arrowsmith
Last modified December 3, 1997