Table for CAN(3,k,13) for k up to 10000

Last Updated Sun Jun 26 08:57:56 MST 2022

Locate the k in the first column that is at least as large as the number of factors in which you are interested. Then let N be the number of rows (tests) given in the second column. A CA(N;3,k,13) exists according to a construction in the reference (cryptically) given in the third column. The accompanying graph plots N vertically against log k (base 10).

Change t: - + Change v: - + or go to Global Menu.

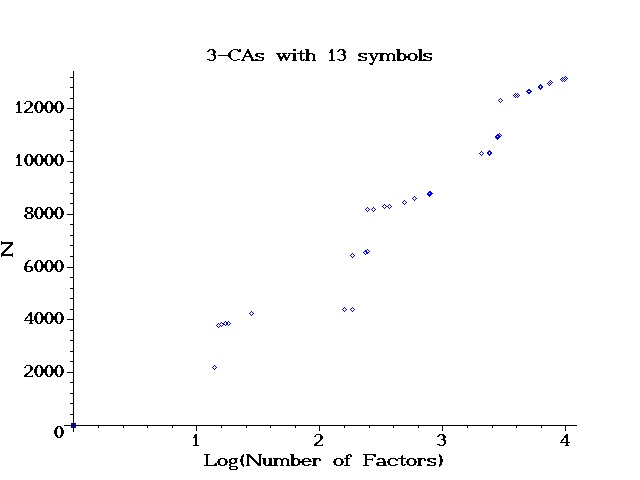
k N Source 14 2197 orthogonal array 15 3798 orthogonal array fuse fuse fuse postop NCK 16 3832 orthogonal array fuse fuse fuse postop NCK 17 3848 orthogonal array fuse fuse fuse postop NCK 18 3863 orthogonal array fuse fuse fuse postop NCK 28 4225 Chateauneuf-Kreher doubling 159 4381 SCPHF LFSR (TJ-IM) 183 4393 Raaphorst-Moura-Stevens 184 6421 Add a factor 235 6565 SCPHF Conditional Expectation (CLS) 242 6589 CPHF Sim Annealing (TJ-IM) 243 8170 SCPHF LFSR (TJ-IM) fuse fuse fuse 273 8185 Raaphorst-Moura-Stevens fuse fuse fuse 338 8281 Path-Restricted SCPHF RE (CLS) 366 8293 Chateauneuf-Kreher doubling 491 8437 Path-Restricted SCPHF RE (CLS) 582 8593 Path-Restricted SCPHF RE (CLS) 773 8749 Restricted CPHF Ext 4( 0, 4) WCS 780 8761 Restricted CPHF Ext 4( 0, 3) WCS 785 8773 Restricted CPHF Ext 4( 0, 2) WCS 791 8785 Restricted CPHF Ext 4( 0, 0) WCS 2067 10309 Colbourn-Martirosyan-TVT-Walker 2068 10320 Cohen-Colbourn-Ling 2379 10321 Colbourn-Martirosyan-TVT-Walker 2380 10332 Cohen-Colbourn-Ling 2758 10933 Restricted CPHF Ext 5( 0, 5) WCS 2794 10945 Restricted CPHF Ext 5( 0, 4) WCS 2823 10957 Restricted CPHF Ext 5( 0, 3) WCS 2855 10969 Restricted CPHF Ext 5( 0, 2) WCS 2904 10981 Restricted CPHF Ext 5( 0, 0) WCS 2925 12337 Restricted CPHF Ext 6( 5, 6) WCS 3858 12493 Restricted CPHF Ext 6( 4, 6) WCS 4005 12505 Restricted CPHF Ext 6( 4, 5) WCS 4978 12649 Restricted CPHF Ext 6( 3, 6) WCS 5029 12661 Restricted CPHF Ext 6( 3, 5) WCS 5086 12673 Restricted CPHF Ext 6( 3, 4) WCS 6159 12805 Restricted CPHF Ext 6( 2, 6) WCS 6170 12817 Restricted CPHF Ext 6( 2, 5) WCS 6244 12829 Restricted CPHF Ext 6( 2, 4) WCS 6266 12841 Restricted CPHF Ext 6( 2, 3) WCS 7295 12961 Restricted CPHF Ext 6( 1, 6) WCS 7365 12973 Restricted CPHF Ext 6( 1, 5) WCS 7396 12985 Restricted CPHF Ext 6( 1, 4) WCS 7461 12997 Restricted CPHF Ext 6( 1, 3) WCS 7469 13009 Restricted CPHF Ext 6( 1, 2) WCS 9393 13117 Restricted CPHF Ext 6( 0, 6) WCS 9753 13129 Restricted CPHF Ext 6( 0, 5) WCS 9938 13141 Restricted CPHF Ext 6( 0, 4) WCS 10000 13153 Restricted CPHF Ext 6( 0, 3) WCS

Graph: