Table for CAN(3,k,19) for k up to 10000

Last Updated Thu Jan 5 06:12:57 MST 2012

Locate the k in the first column that is at least as large as the number of factors in which you are interested. Then let N be the number of rows (tests) given in the second column. A CA(N;3,k,19) exists according to a construction in the reference (cryptically) given in the third column. The accompanying graph plots N vertically against log k (base 10).

Change t: - + Change v: - + or go to Global Menu.

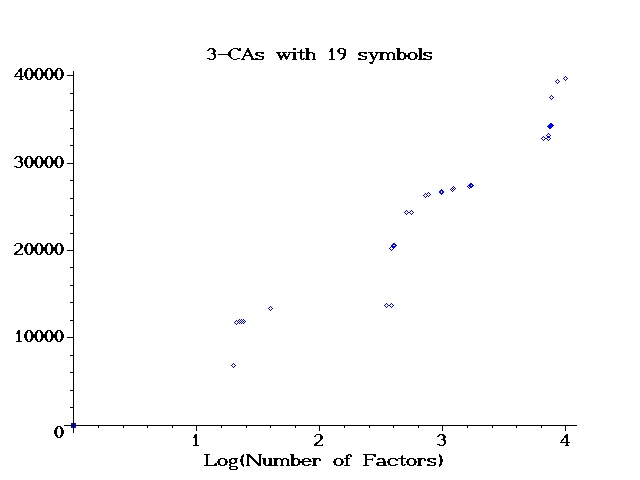
k N Source 20 6859 orthogonal array 21 11838 orthogonal array fuse fuse fuse fuse postop NCK 22 11863 orthogonal array fuse fuse fuse fuse postop NCK 23 11889 orthogonal array fuse fuse fuse fuse postop NCK 24 11927 orthogonal array fuse fuse fuse fuse postop NCK 40 13357 Chateauneuf-Kreher doubling 380 19855 Colbourn-Martirosyan-Trung-Walker 400 25992 Cohen-Colbourn-Ling 418 27343 Colbourn-Martirosyan-Trung-Walker 437 27369 Colbourn-Martirosyan-Trung-Walker 456 27407 Colbourn-Martirosyan-Trung-Walker 475 28891 Colbourn-Martirosyan-Trung-Walker 494 28927 Colbourn-Martirosyan-Trung-Walker 513 28981 Colbourn-Martirosyan-Trung-Walker 532 29017 Colbourn-Martirosyan-Trung-Walker 551 30151 Colbourn-Martirosyan-Trung-Walker 570 30187 Colbourn-Martirosyan-Trung-Walker 589 30223 Colbourn-Martirosyan-Trung-Walker 608 30241 Colbourn-Martirosyan-Trung-Walker 627 31321 Colbourn-Martirosyan-Trung-Walker 646 31339 Colbourn-Martirosyan-Trung-Walker 665 31375 Colbourn-Martirosyan-Trung-Walker 684 31465 Colbourn-Martirosyan-Trung-Walker 760 31735 Colbourn-Martirosyan-Trung-Walker 800 33212 Cohen-Colbourn-Ling 1802 36038 Cyclotomy (Colbourn) fuse 1861 37218 Cyclotomy (Colbourn) fuse 7220 39007 Colbourn-Martirosyan-Trung-Walker 7581 45144 Colbourn-Martirosyan-Trung-Walker 7600 45450 Colbourn-Martirosyan-Trung-Walker 7942 47809 Colbourn-Martirosyan-Trung-Walker 8303 47835 Colbourn-Martirosyan-Trung-Walker 8664 47873 Colbourn-Martirosyan-Trung-Walker 10000 48006 Power N-CT19^4Tlev

Graph: