Table for CAN(5,k,21) for k up to 10000

Last Updated Fri Sep 20 11:41:02 MST 2013

Locate the k in the first column that is at least as large as the number of factors in which you are interested. Then let N be the number of rows (tests) given in the second column. A CA(N;5,k,21) exists according to a construction in the reference (cryptically) given in the third column. The accompanying graph plots N vertically against log k (base 10).

Change t: - + Change v: - + or go to Global Menu.

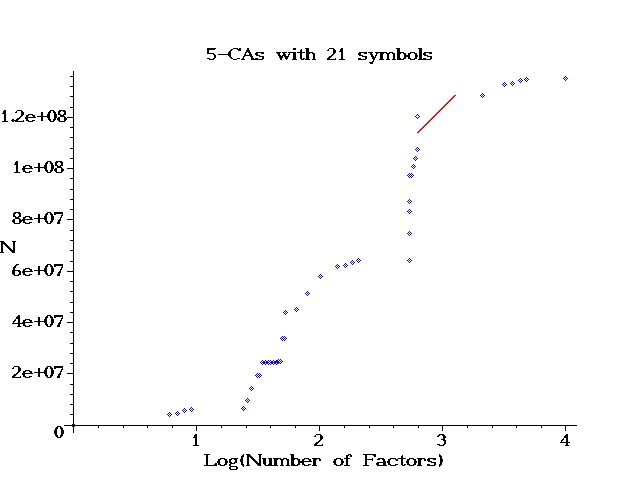
k N Source 6 4084101 Derive from strength 6 7 4473063 Ji-Li-Yin 8 5514335 Add a symbol 9 6094791 Add a symbol 24 6436339 orthogonal array fuse fuse 26 9765617 orthogonal array fuse fuse fuse fuse 28 14348895 orthogonal array fuse fuse fuse fuse fuse fuse 31 19308979 perfect hash family3,31,23,c fuse fuse 32 19309014 perfect hash family3,32,24 34 24465583 Martirosyan-Tran van Trung 36 24475903 Martirosyan-Tran van Trung 38 24512425 Martirosyan-Tran van Trung 40 24524833 Martirosyan-Tran van Trung 42 24559355 Martirosyan-Tran van Trung 44 24566607 Martirosyan-Tran van Trung 46 24610525 Martirosyan-Tran van Trung 48 24682959 Martirosyan-Tran van Trung 50 33716691 Martirosyan-Tran van Trung 52 33816629 Martirosyan-Tran van Trung 53 44034809 AZ1 64 45054259 perfect hash family7,64,23,c fuse fuse 65 45054366 perfect hash family7,65,24 79 51490579 perfect hash family8,79,23,c fuse fuse 80 51490704 perfect hash family8,80,24 101 57926899 perfect hash family9,101,23,c fuse fuse 102 57927042 perfect hash family9,102,24 138 62011142 Power N-CT23^2T17 161 62400104 Power N-CT23^2T16 184 63441376 Power N-CT23^2T15 207 64021832 Power N-CT23^2T14 530 64363380 Power N-CT23^2+1 531 74581560 AZ1 532 83225160 Add 2 factors 533 87114780 Add 3 factors 534 97332960 Add 3 factors 553 97433942 Power CT25^2Arc(3) 576 100763220 Power CT25^2T1T1 600 104092498 Power CT25^2T1 625 107421617 perfect hash family11,625,25,c fuse fuse fuse fuse 626 107421776 Power CT25^2+1 627 120542996 AZ1 2116 128726760 Power CT23^3Tlev 3174 132810860 Power CT23^3T17 3703 133199822 Power CT23^3T16 4232 134241094 Power CT23^3T15 4761 134821550 Power CT23^3T14 10000 135162739 perfect hash family21,12167,23,c fuse fuse

Graph: