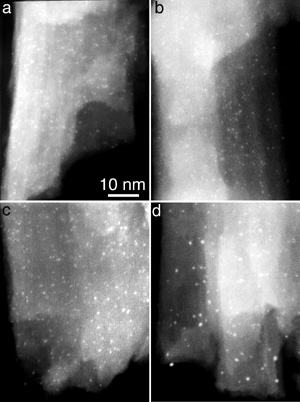
HIGHLIGHTS OF MIKE TREACY'S PAST RESEARCH

The following attempts to encapsulate some of the research projects I have worked on over the years.
 

Proposed and demonstrated the high-angle annular detector for STEM Z contrast


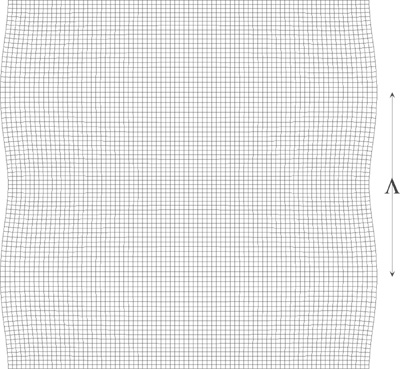My Ph.D. work with Archie Howie was concerned with the characterization of supported Pt and Pd catalysts. Crewe's Z contrast technique seemed ideal for detecting high atomic number (Z) elements such as Pt, on low atomic number supports such as gamma-alumina. However, diffraction into the annular detector produced stronger contrast effects than the simple Rutherford scattering formula predicted. By enlarging the hole in the annular detector, low-angle diffraction is avoided, and the Z-dependence of the signal increases to Z2 because atomic screening effects are diminished. The high-angle detector is now the de-facto standard configuration for Z-contrast studies of materials.

 

Highlighted the importance of elastic relaxation as a source of contrast in modulated thin films
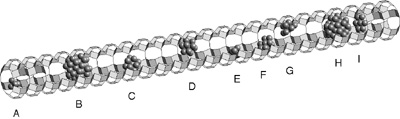My work at CNET in Paris was on spinodal decomposition of InGaAsP semi-conductors which are used as photodiodes in fiber-optic telecommunications. Electron microscopy revealed pronounced quasi-periodic image contrasts which were traditionally ascribed to scattering fluctuations due to local composition changes. In collaboration with J. M. Gibson and A. Howie, I showed that the contrast is primarily due to the bending of lattice planes near surfaces, which is induced by relaxation of strains arising from the modulation in unit cell dimensions. Such bending produces strong diffraction contrasts. Equations for the bending were derived, which remain useful for studies of strain modulation in thin films.

 

Identified a new deactivation mechanism in Pt/K-zeolite L aromatization catalysts


My STEM Z contrast studies of Pt particles in the one-dimensional channels of zeolite L revealed that Pt particles agglomerate slowly with reaction time. The particles remain sufficiently small that over 90% of the Pt atoms reside on particle surfaces. However, double-blockages in the zeolite channels effectively entomb a significant channel volume, and the loss of active Pt can be severe. I proposed a length-loading criterion for maintaining activity. The criterion is simple: there should not be enough Pt per channel to form two or more significant blockages. This hypothesis was confirmed when zeolite L supports with shorter channel lengths, but identical Pt loading, were tested. For proprietary reasons this work (1982–1985), which represents the culmination of my early Z contrast work, was published only in 1999. I was awarded the prestigious Barrer Award (awarded triennially by the British Zeolite Association) in 1990 for part of this work.

 

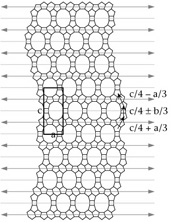Unraveled the structure of zeolite beta


Synthetic zeolite beta was first reported by Mobil in the mid 1960s. Its structure remained a mystery for over 20 years. The presence of planar faults in the sub-micron sized crystallites made it essentially impossible to solve the structure by conventional structure-refining methods. Using TEM to extract structure projections and the symmetry elements, in collaboration with John Newsam, I showed that the structure comprises intimately intergrown right- and left-handed variants of a chiral tetragonal framework. (It later transpired that J. B. Higgins at Mobil had solved the structure prior to me by model-building, but had not been allowed to publish.) The zeolite beta structure is important because it is a 3-dimensional 12-ring framework, with helical channels running along the c-axis. Nobody has synthesized the pure right- or left-handed forms yet, but such a pure end-member structure may have applications in chiral separations. This work paved the way for structure determinations of other intergrown zeolite families.

 

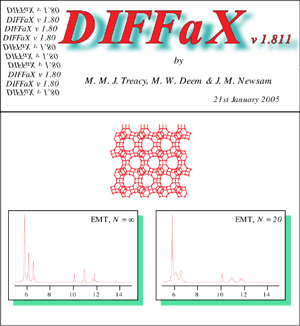
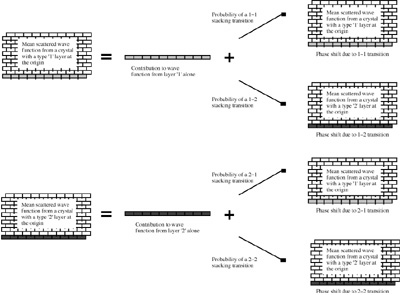Developed recursion algorithm for computing diffraction from faulted crystals

During the course of the zeolite beta work, I developed a method of computing powder x-ray diffraction patterns in the presence of planar faults. The method exploits recursion and is well-adapted to computer implementation. I am the primary author of the fortran computer program DIFFaX that has now been used by hundreds of researchers to simulate diffraction in planar-faulted crystals. The DIFFaX source code, with manual, is supplied free of charge.

 

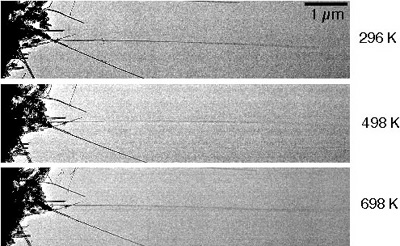Use of thermal vibrations to measure Young's modulus of carbon nanotubes


Long carbon nanotubes that extend over holes in a TEM support film, cannot be imaged clearly at their tips because of vibrations. The vibration amplitude can be several nanometers. In high resolution TEM studies, this motion is normally a problem. I realized that the vibrations are elastically-relaxed phonons and represent heat motion. By measuring the r.m.s. vibration amplitude as a function of temperature I estimated the Young's modulus to be ~1.8 teraPascal, which makes carbon nanotubes the stiffest known material. This unique application of TEM attracted a lot of attention, including highlights in Physics Today, C&E News, New Scientist, Bild der Wissenschaft etc

 

Combinatorial computer method for enumerating zeolite frameworks


In collaboration with computer scientists Randall and Rao, I built a computer program to search combinatorially over every possible crystallographic graph in order to extract all of the 4-connected periodic graphs. For one unique tetrahedral atom there over 6,400 4-connected graphs, of which about 200 refine to regular tetrahedral topology. This work took over 10 years to bring to fruition, and discovered many new theoretical zeolite frameworks, and revealed some interesting idiosyncrasies in the International Tables for Crystallography.

 

Developed the Fluctuation Microscopy Technique: A probe of medium range order in amorphous materials


In collaboration with Murray Gibson, we have shown that the speckle observed in dark-field images of amorphous materials can be used to measure medium-range order. Diffraction techniques are sensitive to pair-correlations, and are most sensitive to short-range order. We showed that the speckle intensity variance is sensitive to 4-body correlations and is therefore sensitive to medium-range order. By using hollow-cone illumination to control the spatial coherence of the illumination, a speckle signature of a sample can be extracted. We have termed this technique, variable coherence microscopy, and it is a form of a new class of analytical techniques we call fluctuation microscopy. Using variable coherence microscopy, we have shown that as-deposited amorphous germanium and silicon contain paracrystalline regions, which on annealing below the recrystallization temperature transform to the lower-energy continuous random network. We have also shown that amorphous hydrogenated silicon (a-Si:H) undergoes a significant structural re-arrangement on light-soaking. We hope that this latter observation may lead to an improved understanding of the Staebler-Wronski effect, where a-Si:H photovoltaic detectors lose efficiency on prolonged exposure to sunlight, a fact that currently limits the efficiency of a-Si:H solar cells.

 

Characterization of stacking fault patterns in faujasitic zeolites using TEM and DIFFaX simulations

The tools I developed for studying zeolite beta where applied to studying the faulting distributions in the various faujasite-related synthetic zeolites, ranging from pure cubic FAU framework to the pure hexagonal EMT framework. Using TEM and DIFFaX, I showed that the faulting in these materials is clustered. Using the strain relaxation model, I showed that the strains associated with the stacking faults were reduced when faults were clustered. I won the prestigious Breck Award (awarded triennially by the International Zeolite Association) in 1996 for this work.

 

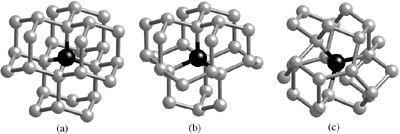Schläfli Cluster methods for modeling amorphous tetrahedral models

Borrowing from my work on zeolite topologies, I have developed a simple topological tool for investigating medium-range order in models of amorphous tetrahedral semiconductors. Schläfli clusters are compact topological descriptors of the local connectivity around each atom. (It later emerged that they are similar to the earlier “local cluster” concept of L. W. Hobbs et al.) I have proposed that the diamond Schläfli cluster is the minimum atomic configuration that can be called “topologically cubic”. Searching for such clusters is a fast effective tool for detecting medium range order in models of amorphous semiconductors. This work is in progress.

 

In-situ TEM observations of domain switching in ferroelectric thin films.

In collaboration with A. Krishnan, we made in-situ TEM observations of domain wall motion in thin single crystal ferroelectric materials under applied electric fields. I designed, and had built, a special TEM specimen holder that can heat, apply electric fields and shine light onto a sample. Our observations showed that domain walls do not move as rigid membranes. Instead, we proposed that domain walls move by allowing charged ripples to propagate along them. We developed a simple Landau-Ginsburg Free energy argument showing that ripples have a reduced barrier to switching. Ripples enable wall motion by a mechanism analogous to that for dislocation motion in crystal slip. We also showed that some domain walls are locked under certain electric field directions, representing an inherent contribution to ferroelectric fatigue and imprint.
 

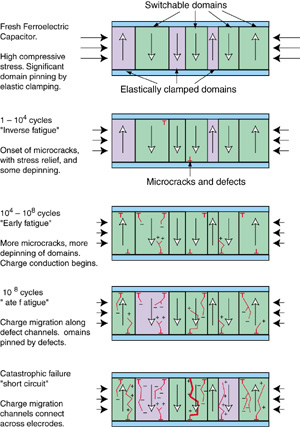Developed a model of fatigue in thin-film PZT ferroelectrics.

In collaboration with postdoctoral student Kitae Park, and Shobo Bhattacharya at NECI, Princeton, we developed a simple two-stage model of ferroelectric fatigue in thin films of PZT sandwiched between Pt electrodes and supported on Si. Such devices are used in fast, low-power, non-volatile memories. By a combination of electrical measurements, cross-section TEM and modelling, we showed that; (i) Early in the life of a ferroelectric, stresses between the electrodes and PZT cause microcracking at the interface. This acts to weaken the applied electric fields (because of the air gap effect), and sets the stage for the the next step. (ii) Later, defects extending into the PZT layers screen the applied electric fields, inhibiting the ferroelectric switching, and eventually leading to short circuits. A thin conducting (non-ferroelectric) oxide layer between the PZT and electrodes almost eliminates fatigue completely by acting as a buffer layer for elastic stresses, and providing a stronger bonding between the electrode and the ferroelectric itself. We developed a two-parameter phenomenological model that modelled the decay, and which allowed the prediction of device fatigue behavior as a function of temperature and applied voltage.

 

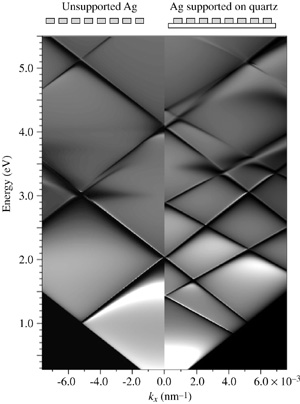Developed an effective dynamical diffraction Bloch wave explanation for the anomalous transmission of light through thin hole arrays.

When light is shone on a thin metallic film, which has a periodic array of sub-optical wavelength diameter holes drilled through it, anomalously high intensities are transmitted at certain wavelengths. That is, more light gets through than would be expected from the hole area. The current popular explanation is that surface plasmons “guide” the light through the holes. I have developed an alternative dynamical diffraction Bloch wave theory that completely explains the anomalous transmission, and does so without resorting to special pleading about surface plasmons. The theory is fully general for 3-dimensional periodic gratings, and unlike the other theories, makes no simplifications or approximations to Maxwell’s equations.

 

Designability of graphitic carbon cones.

In collaboration with Ebbesen, Krishnan and DuJardin, we described in the journal Nature a special carbon black sample that comprised a high density of graphitic disks and cones. Our TEM analysis confirmed that the five topologically-allowed conical forms all occur, but with a preponderance of the 60° cone-angle variety. I explain this distribution with a simple model of graphitization. I point out that there are many more ways to circumscribe carbon rings around the tip of a cone than there are ways to imbed the same rings in planar graphite. For topologically flexible seeds, graphitic cones are more “designable” than planar graphite. With an assumed seed distribution, the model explains the observed cone distribution – highlighting the role of entropy in the formation of curved graphitic structures.


Back to Mike Treacy's homepage
Last updated July 2nd, 2005.

You are visitor number