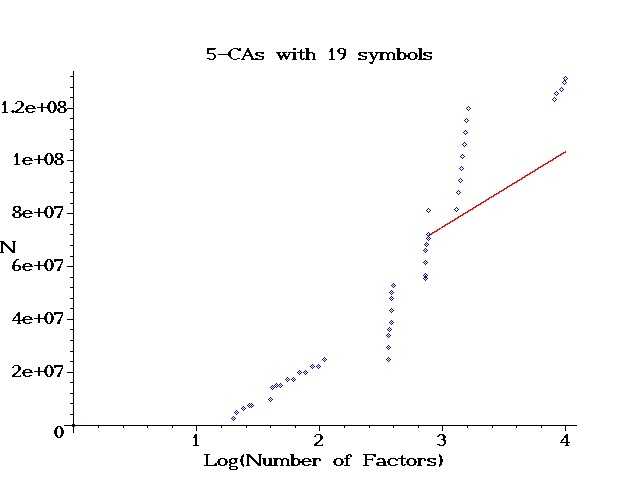
| k | N | Source |
| 20 | 2476099 | orthogonal array |
| 21 | 4815018 | extended OA (Colbourn) |
| 24 | 6436335 | orthogonal array fuse fuse fuse fuse |
| 27 | 7428259 | perfect hash family3,27,19,c |
| 28 | 7428294 | perfect hash family3,28,20 |
| 40 | 9774075 | Martirosyan-TVT |
| 41 | 14342169 | Add 1 factors |
| 44 | 14856499 | perfect hash family6,44,19,c |
| 48 | 14856588 | perfect hash family6,48,20 |
| 55 | 17332579 | perfect hash family7,55,19,c |
| 61 | 17332686 | perfect hash family7,61,20 |
| 68 | 19808659 | perfect hash family8,68,19,c |
| 76 | 19808784 | perfect hash family8,76,20 |
| 87 | 22284739 | perfect hash family9,87,19,c |
| 98 | 22284882 | perfect hash family9,98,20 |
| 108 | 24760819 | perfect hash family10,108,19,c |
| 362 | 24760980 | Power N-CT19^2+1 |
| 363 | 29452194 | Add 1 factors |
| 364 | 34020288 | Add 2 factors |
| 365 | 36366066 | Add 3 factors |
| 381 | 38711844 | Add 19 factors |
| 382 | 43403058 | Add 19 factors |
| 383 | 47971152 | Add 19 factors |
| 384 | 50316930 | Add 19 factors |
| 400 | 52662708 | Add 19 factors |
| 722 | 55399088 | Martirosyan-TVT |
| 724 | 56685692 | Martirosyan-TVT |
| 726 | 61376906 | Martirosyan-TVT |
| 728 | 65945000 | Martirosyan-TVT |
| 730 | 68290778 | Martirosyan-TVT |
| 760 | 70636556 | Martirosyan-TVT |
| 762 | 72188534 | Martirosyan-TVT |
| 763 | 81207416 | Add 1 factors |
| 1284 | 81631477 | Power N-CT41^2Arc(9)T2 |
| 1348 | 87966666 | Power N-CT41^2Arc(9) |
| 1381 | 92534760 | Power N-CT41^2Arc(8) |
| 1415 | 97102854 | Power N-CT41^2Arc(7) |
| 1450 | 101670948 | Power N-CT41^2Arc(6) |
| 1486 | 106239042 | Power N-CT41^2Arc(5) |
| 1523 | 110807136 | Power N-CT41^2Arc(4) |
| 1561 | 115375230 | Power N-CT41^2Arc(3) |
| 1600 | 119943324 | Power N-CT41^2T1T1 |
| 8000 | 123282306 | Power CT23^3T3T3T3 |
| 8400 | 125621225 | Power CT23^3T3T3T2 |
| 9200 | 127242542 | Power CT23^3T3T3 |
| 9660 | 129581461 | Power CT23^3T3T2 |
| 10000 | 131202778 | Power CT23^3T3 |
| | Graph:
 |
