Table for CAN(6,k,22) for k up to 10000

Last Updated Fri Sep 20 11:45:18 MST 2013

Locate the k in the first column that is at least as large as the number of factors in which you are interested. Then let N be the number of rows (tests) given in the second column. A CA(N;6,k,22) exists according to a construction in the reference (cryptically) given in the third column. The accompanying graph plots N vertically against log k (base 10).

Change t: - Change v: - + or go to Global Menu.

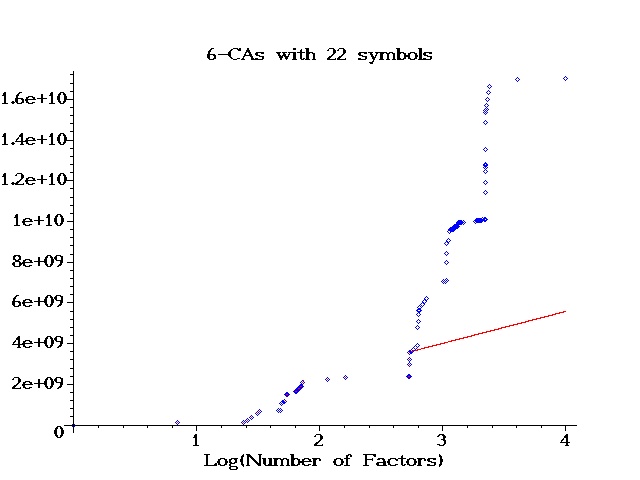
k N Source 7 113379904 composition 24 148035887 orthogonal array fuse 26 244140619 orthogonal array fuse fuse fuse 28 387420479 orthogonal array fuse fuse fuse fuse fuse 31 592143485 perfect hash family4,31,23,c fuse 32 688248276 perfect hash family4,35,25S3 46 714434071 double OA (Colbourn-Zhou) fuse 48 720858247 double OA (Colbourn-Zhou) fuse 49 1040941349 Add 23 factors 50 1102986371 Martirosyan-Tran van Trung 52 1166266649 Martirosyan-Tran van Trung 54 1480358681 perfect hash family10,54,23,c fuse 55 1480358860 perfect hash family10,55,24 63 1628394547 perfect hash family11,63,23,c fuse 64 1628394746 perfect hash family11,64,24 67 1776430413 perfect hash family12,67,23,c fuse 68 1776430632 perfect hash family12,68,24 69 1903843237 Add 23 factors 71 1910267413 Add 23 factors 72 2072502404 perfect hash family14,72,24 115 2220538011 perfect hash family15,115,23,c fuse 161 2333918193 Power CT23^2T16 529 2368573877 perfect hash family16,529,23,c fuse 530 2368574176 Power CT23^2+1 531 2997898102 Add 1 factors 532 3245198386 Add 2 factors 533 3565308792 Add 3 factors 553 3617935692 Power CT25^2Arc(3) 576 3714040424 Power CT25^2T1T1 600 3810145156 Power CT25^2T1 625 3906249619 perfect hash family16,625,25,c fuse fuse fuse 626 3906249888 Power CT25^2+1 627 4769477484 Add 1 factors 628 5087031336 Add 2 factors 629 5444936730 Add 3 factors 630 5616304910 Add 5 factors 648 5650960895 Add 23 factors 651 5768888068 Power CT27^2Arc(3) 676 5912167928 Power CT27^2T1T1 702 6055447788 Power CT27^2T1 730 6198727648 Power CT27^2+1 1008 7061067952 Martirosyan-Tran van Trung 1058 7063792298 Martirosyan-Tran van Trung 1060 7112753357 Martirosyan-Tran van Trung 1062 7978136645 Martirosyan-Tran van Trung 1064 8441015369 Martirosyan-Tran van Trung 1066 8917476739 Martirosyan-Tran van Trung 1104 9056596717 Martirosyan-Tran van Trung 1106 9081079117 Martirosyan-Tran van Trung 1128 9519193084 Power N-CT47^2T23 1147 9596025288 Power N-CT47^2Arc(3)T21 1171 9602449464 Power N-CT47^2Arc(2)T21 1196 9608873640 Power N-CT47^2T21T1 1222 9615297816 Power N-CT47^2T21 1235 9739305148 Power N-CT47^2Arc(3)T19 1261 9745729324 Power N-CT47^2Arc(2)T19 1288 9752153500 Power N-CT47^2T19T1 1316 9758577676 Power N-CT47^2T19 1333 9879786394 Power N-CT43^2T12 1339 9937603978 Power N-CT47^2Arc(4)T16 1367 9944028154 Power N-CT47^2Arc(3)T16 1396 9950452330 Power N-CT47^2Arc(2)T16 1426 9956876506 Power N-CT47^2T16T1 1457 9963300682 Power N-CT47^2T16 1852 10002076980 Power N-CT43^2+3 1861 10040622036 Power N-CT47^2Arc(8) 1901 10047046212 Power N-CT47^2Arc(7) 1942 10053470388 Power N-CT47^2Arc(6) 1984 10059894564 Power N-CT47^2Arc(5) 2027 10066318740 Power N-CT47^2Arc(4) 2071 10072742916 Power N-CT47^2Arc(3) 2116 10079167092 Power N-CT47^2T1T1 2162 10085591268 Power N-CT47^2T1 2210 10092015444 Power N-CT47^2+1 2211 11417634816 Add 1 factors 2212 11934616512 Add 2 factors 2213 12458314192 Add 3 factors 2214 12659945204 Add 4 factors 2215 12766888765 Add 5 factors 2233 12801544750 Add 23 factors 2235 13561684044 Add 25 factors 2236 14887303416 Add 25 factors 2237 15369474510 Add 23 factors 2238 15476418071 Add 23 factors 2256 15511074056 Add 23 factors 2257 15694812262 Power CT49^2Arc(3) 2304 16014895364 Power CT49^2T1T1 2352 16334978466 Power CT49^2T1 2401 16655061568 Power CT49^2 4032 16989469227 Power CT31^3T24T7T7 10000 17024125210 Power CT31^3T13T7T7

Graph: