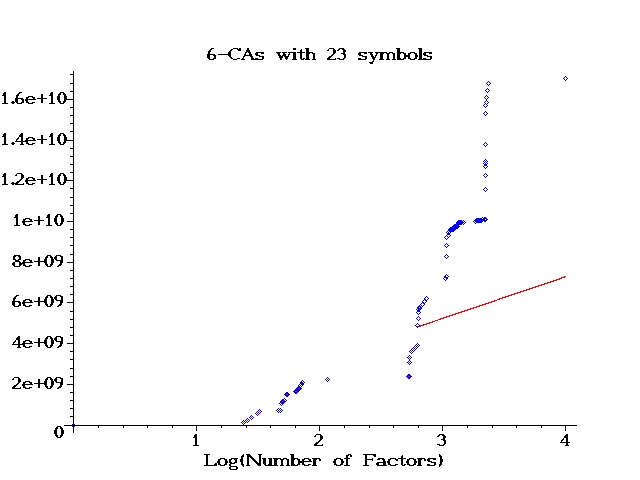
| k | N | Source |
| 24 | 148035889 | orthogonal array |
| 26 | 244140621 | orthogonal array fuse fuse |
| 28 | 387420481 | orthogonal array fuse fuse fuse fuse |
| 31 | 592143487 | perfect hash family4,31,23,c |
| 32 | 688248284 | perfect hash family4,35,25S3 |
| 46 | 714434073 | double OA (Colbourn-Zhou) |
| 48 | 720858249 | double OA (Colbourn-Zhou) |
| 49 | 1071585217 | Add 23 factors |
| 50 | 1181640621 | double OA (Colbourn-Zhou) fuse fuse |
| 52 | 1183995124 | Martirosyan-Tran van Trung |
| 54 | 1480358683 | perfect hash family10,54,23,c |
| 55 | 1480358880 | perfect hash family10,55,24 |
| 63 | 1628394549 | perfect hash family11,63,23,c |
| 64 | 1628394768 | perfect hash family11,64,24 |
| 67 | 1776430415 | perfect hash family12,67,23,c |
| 68 | 1776430656 | perfect hash family12,68,24 |
| 69 | 1924466544 | perfect hash family13,69,24 |
| 70 | 1966334239 | Add 23 factors |
| 71 | 1972223067 | Add 23 factors |
| 72 | 2072502432 | perfect hash family14,72,24 |
| 115 | 2220538013 | perfect hash family15,115,23,c |
| 529 | 2368573879 | perfect hash family16,529,23,c |
| 530 | 2368574208 | Power CT23^2+1 |
| 531 | 3057834758 | Add 1 factors |
| 532 | 3341022212 | Add 2 factors |
| 553 | 3617935724 | Power CT25^2Arc(3) |
| 576 | 3714040456 | Power CT25^2T1T1 |
| 600 | 3810145188 | Power CT25^2T1 |
| 625 | 3906249621 | perfect hash family16,625,25,c fuse fuse |
| 626 | 3906249920 | Power CT25^2+1 |
| 627 | 4870494146 | Add 1 factors |
| 628 | 5234123456 | Add 2 factors |
| 629 | 5534989032 | Add 3 factors |
| 630 | 5676588578 | Add 4 factors |
| 648 | 5708143451 | Add 23 factors |
| 651 | 5768888100 | Power CT27^2Arc(3) |
| 676 | 5912167960 | Power CT27^2T1T1 |
| 702 | 6055447820 | Power CT27^2T1 |
| 730 | 6198727680 | Power CT27^2+1 |
| 1058 | 7196366258 | Martirosyan-Tran van Trung |
| 1060 | 7316819887 | Martirosyan-Tran van Trung |
| 1062 | 8276955393 | Martirosyan-Tran van Trung |
| 1064 | 8825140107 | Martirosyan-Tran van Trung |
| 1066 | 9237496663 | Martirosyan-Tran van Trung |
| 1104 | 9293733855 | Martirosyan-Tran van Trung |
| 1106 | 9450211365 | Martirosyan-Tran van Trung |
| 1128 | 9519193112 | Power N-CT47^2T23 |
| 1147 | 9596025316 | Power N-CT47^2Arc(3)T21 |
| 1171 | 9602449492 | Power N-CT47^2Arc(2)T21 |
| 1196 | 9608873668 | Power N-CT47^2T21T1 |
| 1222 | 9615297844 | Power N-CT47^2T21 |
| 1235 | 9739305176 | Power N-CT47^2Arc(3)T19 |
| 1261 | 9745729352 | Power N-CT47^2Arc(2)T19 |
| 1288 | 9752153528 | Power N-CT47^2T19T1 |
| 1316 | 9758577704 | Power N-CT47^2T19 |
| 1333 | 9879786422 | Power N-CT43^2T12 |
| 1339 | 9937604006 | Power N-CT47^2Arc(4)T16 |
| 1367 | 9944028182 | Power N-CT47^2Arc(3)T16 |
| 1396 | 9950452358 | Power N-CT47^2Arc(2)T16 |
| 1426 | 9956876534 | Power N-CT47^2T16T1 |
| 1457 | 9963300710 | Power N-CT47^2T16 |
| 1852 | 10002077008 | Power N-CT43^2+3 |
| 1861 | 10040622064 | Power N-CT47^2Arc(8) |
| 1901 | 10047046240 | Power N-CT47^2Arc(7) |
| 1942 | 10053470416 | Power N-CT47^2Arc(6) |
| 1984 | 10059894592 | Power N-CT47^2Arc(5) |
| 2027 | 10066318768 | Power N-CT47^2Arc(4) |
| 2071 | 10072742944 | Power N-CT47^2Arc(3) |
| 2116 | 10079167120 | Power N-CT47^2T1T1 |
| 2162 | 10085591296 | Power N-CT47^2T1 |
| 2210 | 10092015472 | Power N-CT47^2+1 |
| 2211 | 11593923154 | Add 1 factors |
| 2212 | 12277550912 | Add 2 factors |
| 2213 | 12690036546 | Add 3 factors |
| 2214 | 12831636092 | Add 4 factors |
| 2233 | 12973235638 | Add 23 factors |
| 2235 | 13781923672 | Add 25 factors |
| 2236 | 15283831354 | Add 25 factors |
| 2237 | 15712856258 | Add 23 factors |
| 2256 | 15854455804 | Add 23 factors |
| 2257 | 16093182552 | Power CT49^2Arc(3) |
| 2304 | 16443909520 | Power CT49^2T1T1 |
| 2352 | 16794636488 | Power CT49^2T1 |
| 10000 | 17024125272 | Power CT31^3T13T7T7 |